We don't have a formula for y. We could solve this equation for y, but we would find 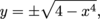
which is two equations. We would then take two separate derivatives, and that's too much work. Instead, take derivatives of both sides of the equation, with respect to x: 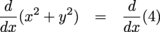
Since the derivative of a sum is the sum of the derivatives, and the derivative of a constant is 0, 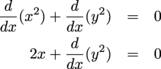
Pause for a minute. While we don't have a formula for y, we know that y is a function of x. That is, y is the inside function and (□)2 is the outside function. To take the derivative of y2 we need to use the chain rule: 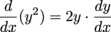
Put this back in the equation where we left off: 
It's like magic. In the original equation we had a messy y2 term, but now we have dy/dx. Solving for the derivative, we find 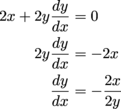
In this type of problem, it's fine to have y in the definition of the derivative. We can't write y in terms of x since we don't have a formula for y in the first place. The final answer is 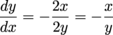
|