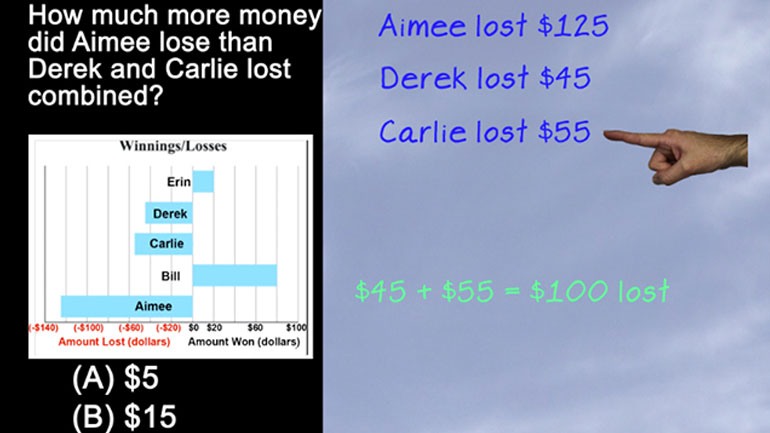
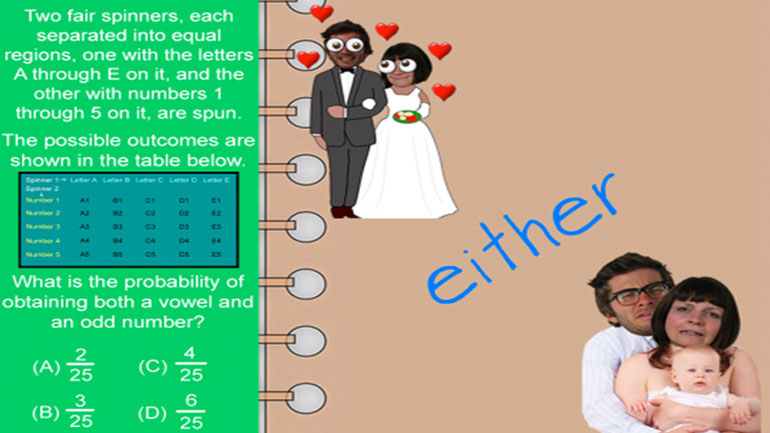
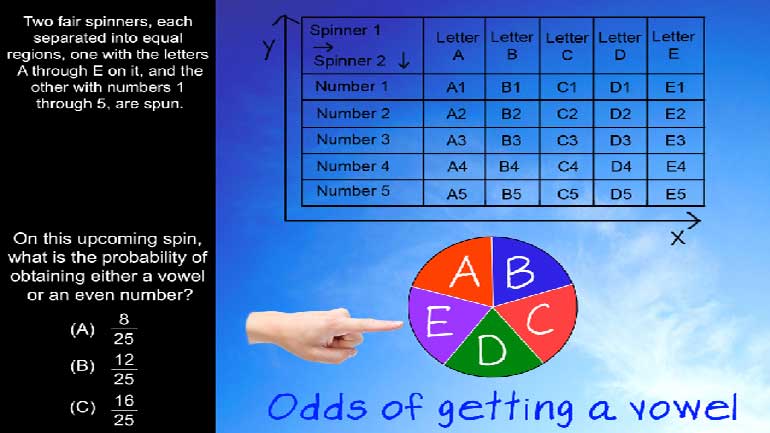
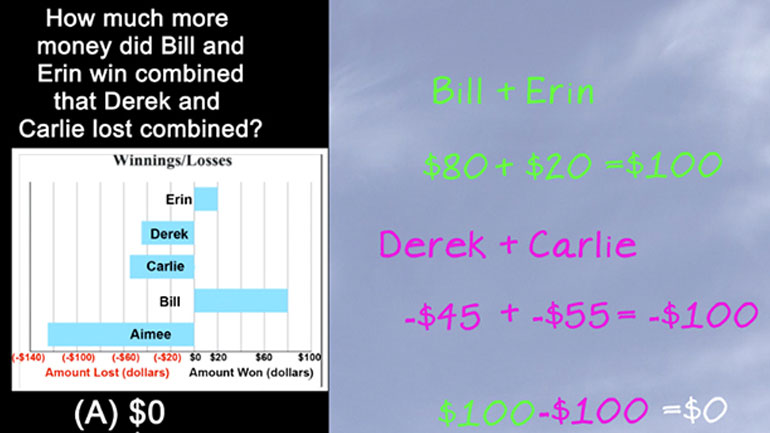
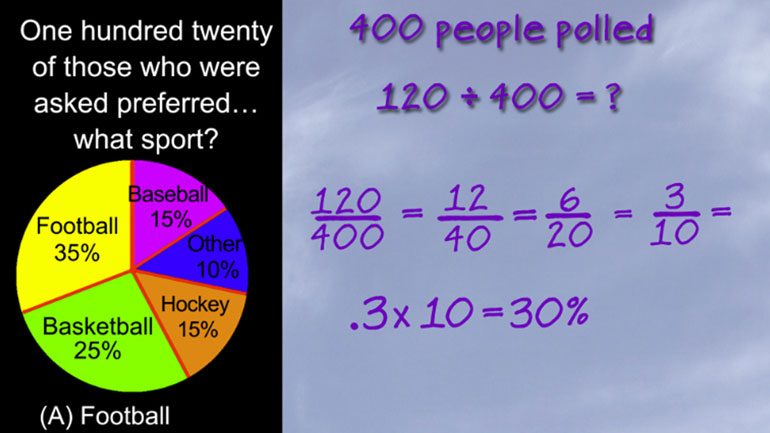
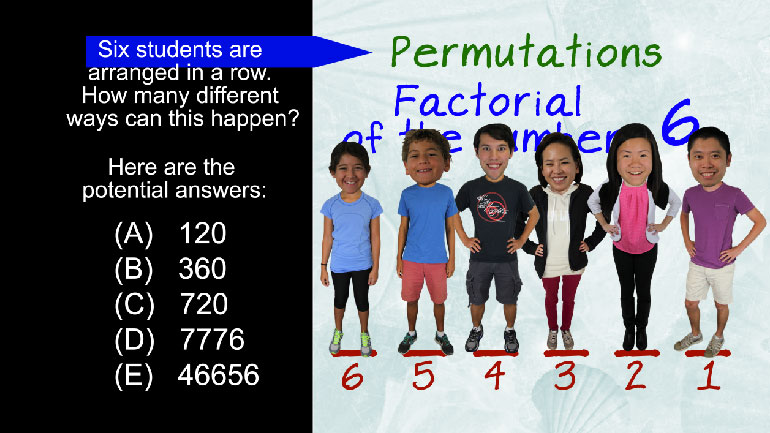
ShmoopTube
Where Monty Python meets your 10th grade teacher.
Search Thousands of Shmoop Videos
Statistics and Probability Videos 134 videos
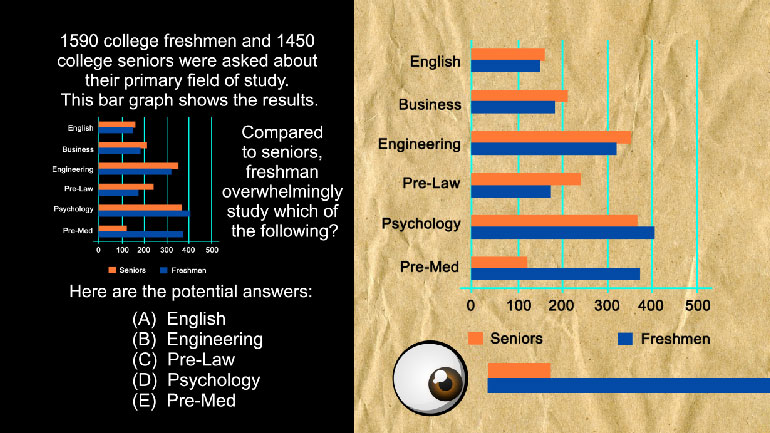
SAT Math: Statistics and Probability Drill 1, Problem 2. If the four largest numbers in the set were doubled, what would happen to the median...
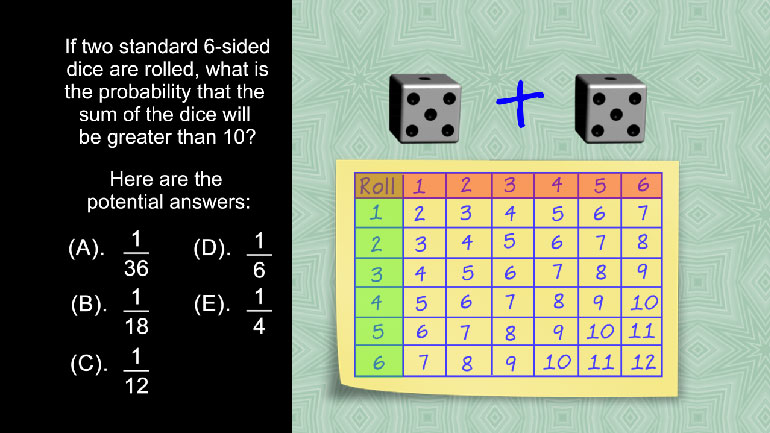
SAT Math 1.4 Statistics and Probability. If two standard 6-sided dice are rolled, what is the probability that the sum of the dice will be gre...
SAT Math 1.5 Statistics and Probability. If Silas draws one card, then places it in his pocket and draws another, what is the probability that...
CAHSEE Math 5.1 Statistics, Data, and Probability I 174 Views
Share It!
Description:
Statistics, Data, and Probability I: Drill Set 5, Problem 1. When a normal Vegas pair of dice with six sides is tossed, what is the probability that the sum of the numbers will be greater than or equal to 3?
Transcript
- 00:03
Here's a shmoopy question for you...
- 00:05
When a normal Vegas pair of dice with six sides is tossed, what is the probability that
- 00:11
the sum of the numbers will be greater than or equal to 3?
- 00:15
You've already gotten in trouble once for sneaking in a pair of your weighted dice,
- 00:19
so we know you wouldn't dare pulling that stunt again.
Full Transcript
- 00:22
And here are the potential answers...
- 00:27
OK, so what is this question really asking?
- 00:30
Well, it's a two-parter -- that is, 2 events happen and we have to figure out the odds
- 00:34
of the number from roll 1 plus the number from roll 2 being greater than or equal to 3.
- 00:40
If we just think about this a moment before doing ANY math, the SUM of these
- 00:45
rolls is almost ALWAYS going to be greater than 3 -- like,
- 00:49
the worst we could do is a 1 and a 1 but how many times does that happen?
- 00:53
If we rolled a 2 and a 1, we'd be at least equal to three -- same deal with a 1 and a 2.
- 00:59
Then EVERY OTHER ROLL combo is greater than or equal to 3.
- 01:03
So we KNOW that the odds are going to be VERY
- 01:06
high that we solve the question with a yes... or a true.
- 01:09
And if we just glance at the answer set, we could probably very quickly throw out answers
- 01:13
A and B because those show long odds and ours are going to be... the opposite.
- 01:19
If we had to, we could brute force with this question by laying out all of the 36 combinations
- 01:24
of rolls... like this...
- 01:25
...and counting how many numbers are greater than or equal to 3.
- 01:29
But that's a lotta work and time consuming and doesn't scale -- like... what if they
- 01:32
asked us for 5 dice rolls' odds? Yeah, then we'd be up test creek without a pencil.
- 01:39
So instead, since we know that the odds are going to very high, it'd be easier for us
- 01:44
to count the number of times the sum of dice is less than 3, or equal to 2, since 1 + 1
- 01:49
= 2, and 1 is the lowest number on a dice. We also know that 6 times 6, or 36, tells
- 01:55
us how many possible sum combinations we can get.
- 01:59
Not how many unique sums, but just
- 02:01
how many combinations we could get in total if we really wanted to...
- 02:05
Now we can apply the principle that the probability
- 02:08
of something occurring is the same as 1 minus the probability of that something not occurring.
- 02:14
In math speak, this would be P = 1 - (not P).
- 02:17
In this case, the 'not P' is the probability that the sum of the dice, when rolled,
- 02:22
is 1 over 36, which we found just a few seconds ago.
- 02:26
So, P = 1 - 1/36 equals 35/36.
- 02:31
The correct answer is D.
- 02:34
And yet... cat's eyes. Interesting.
- 02:36
You might not want to keep those dice of yours in your back pocket...
Related Videos
CAHSEE Math: Algebra and Functions Drill 5, Problem 3. Solve the equation.
SAT Math: Statistics and Probability Drill 1, Problem 2. If the four largest numbers in the set were doubled, what would happen to the median...
SAT Math 1.4 Statistics and Probability. If two standard 6-sided dice are rolled, what is the probability that the sum of the dice will be gre...
SAT Math 1.5 Statistics and Probability. If Silas draws one card, then places it in his pocket and draws another, what is the probability that...